|
Two-dimensional Euler's Equations of Gas Dynamics
In this example we use a two-dimensional second order fully-discrete central scheme to evolve the solution of Euler's equations of gas dynamics
where the pressure, p, is related to the conserved quantities through the equation of state
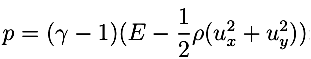
with 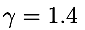 . We solve a two-d Riemann problem over the computational domain . We solve a two-d Riemann problem over the computational domain 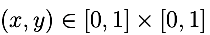 , with initial conditions given by , with initial conditions given by
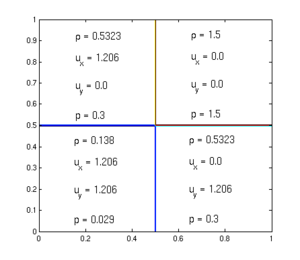
and Dirichlet boundary conditions (i.e., the conserved quantities take on the values specified by the initial conditions at either boundary). The solution is evolved up to 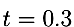 , using a 200 x 200 mesh and cfl number 0.75. , using a 200 x 200 mesh and cfl number 0.75.
The images below display the contour lines of density, and pressure (top) and the velocity field (bottom) at 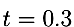 ,. Click on the individual images to see an animation from ,. Click on the individual images to see an animation from 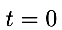 to to 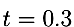 . .
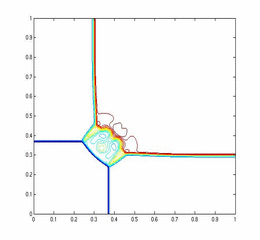 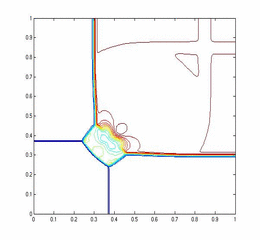
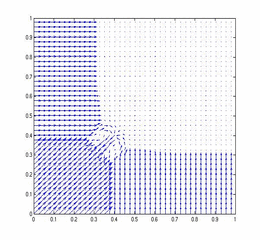
Click on the images above to see an animation
| 
